
Cartan matrix
Encyclopedia
In mathematics
, the term Cartan matrix has three meanings. All of these are named after the French
mathematician
Élie Cartan
. In fact, Cartan matrices in the context of Lie algebra
s were first investigated by Wilhelm Killing
, whereas the Killing form
is due to Cartan.
 with integer
with integer
entries such that
The third condition is not independent but is really a consequence of the first and fourth conditions.
We can always choose a D with positive diagonal entries. In that case,
if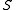 in the above decomposition is positive definite
in the above decomposition is positive definite
, then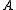 is said to be a Cartan matrix.
is said to be a Cartan matrix.
The Cartan matrix of a simple Lie algebra
is the matrix whose elements are the scalar products
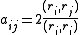
(sometimes called the Cartan integers) where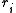 are the simple roots
are the simple roots
of the algebra. The entries are integral from one of the properties of root
s. The first condition follows from the definition, the second from the fact that for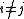 ,
,  is a root which is a linear combination
is a root which is a linear combination
of the simple root
s ri and rj with a positive coefficient for rj and so, the coefficient for ri has to be nonnegative. The third is true because orthogonality is a symmetric relation. And lastly, let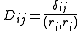 and
and 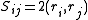 . Because the simple roots span a Euclidean space
. Because the simple roots span a Euclidean space
, S is positive definite.
Conversely, given a generalized Cartan matrix, one can recover its corresponding Lie algebra. (See Kac-Moody algebra for more details).
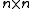 matrix A is decomposable if there exists a nonempty proper subset
matrix A is decomposable if there exists a nonempty proper subset 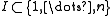 such that
such that 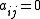 whenever
whenever 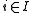 and
and 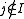 . A is indecomposable if it is not decomposable.
. A is indecomposable if it is not decomposable.
Let A be an indecomposable generalized Cartan matrix. We say that A is of finite type if all of its principal minors are positive, that A is of affine type if its proper principal minors are positive and A has determinant
0, and that A is of indefinite type otherwise.
Finite type indecomposable matrices classify the finite dimensional simple Lie algebras (of types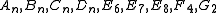 ), while affine type indecomposable matrices classify the affine Lie algebra
), while affine type indecomposable matrices classify the affine Lie algebra
s (say over some algebraically closed field of characteristic 0).
, and more generally in the theory of representations of finite-dimensional associative algebra
s A that are not semisimple
, a Cartan matrix is defined by considering a (finite) set of principal indecomposable module
s and writing composition series
for them in terms of irreducible modules, yielding a matrix of integers counting the number of occurrences of an irreducible module.
, one may consider a geometry with two-cycles
which intersects with each other at a finite number of points, at the limit where the area of the two-cycles go to zero. At this limit, there appears a local symmetry group. The matrix of intersection number
s of a basis of the two-cycles is conjectured to be the Cartan matrix of the Lie algebra
of this local symmetry group http://arxiv.org/abs/hep-th/9707123.
This can be explained as follows. In M-theory one has soliton
s which are two-dimensional surfaces called membranes or 2-branes. A 2-brane has a tension and thus tends to shrink, but it may wrap around a two-cycles which prevents it from shrinking to zero.
One may compactify
one dimension which is shared by all two-cycles and their intersecting points, and then take the limit where this dimension shrinks to zero, thus getting a dimensional reduction
over this dimension. Then one gets type IIA string theory
as a limit of M-theory, with 2-branes wrapping a two-cycles now described by an open string stretched between D-brane
s. There is a U(1) local symmetry group for each D-brane, resembling the degree of freedom
of moving it without changing its orientation. The limit where the two-cycles have zero area is the limit where these D-branes are on top of each other, so that one gets an enhanced local symmetry group.
Now, an open string stretched between two D-branes represents a Lie algebra generator, and the commutator
of two such generator is a third one, represented by an open string which one gets by gluing together the edges of two open strings.
The latter relation between different open strings is dependent on the way 2-branes may intersect in the original M-theory, i.e. in the intersection numbers of two-cycles. Thus the Lie algebra depends entirely on these intersection numbers. The precise relation to the Cartan matrix is because the latter describes the commutators of the simple root
s, which are related to the two-cycles in the basis that is chosen.
Note that generators in the Cartan subalgebra are represented by open strings which are stretched between a D-brane and itself.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, the term Cartan matrix has three meanings. All of these are named after the French
France
The French Republic , The French Republic , The French Republic , (commonly known as France , is a unitary semi-presidential republic in Western Europe with several overseas territories and islands located on other continents and in the Indian, Pacific, and Atlantic oceans. Metropolitan France...
mathematician
Mathematician
A mathematician is a person whose primary area of study is the field of mathematics. Mathematicians are concerned with quantity, structure, space, and change....
Élie Cartan
Élie Cartan
Élie Joseph Cartan was an influential French mathematician, who did fundamental work in the theory of Lie groups and their geometric applications...
. In fact, Cartan matrices in the context of Lie algebra
Lie algebra
In mathematics, a Lie algebra is an algebraic structure whose main use is in studying geometric objects such as Lie groups and differentiable manifolds. Lie algebras were introduced to study the concept of infinitesimal transformations. The term "Lie algebra" was introduced by Hermann Weyl in the...
s were first investigated by Wilhelm Killing
Wilhelm Killing
Wilhelm Karl Joseph Killing was a German mathematician who made important contributions to the theories of Lie algebras, Lie groups, and non-Euclidean geometry....
, whereas the Killing form
Killing form
In mathematics, the Killing form, named after Wilhelm Killing, is a symmetric bilinear form that plays a basic role in the theories of Lie groups and Lie algebras...
is due to Cartan.
Lie algebras
A generalized Cartan matrix is a square matrix with integer
with integerInteger
The integers are formed by the natural numbers together with the negatives of the non-zero natural numbers .They are known as Positive and Negative Integers respectively...
entries such that
- For diagonal entries,
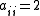 .
. - For non-diagonal entries,
 .
. -
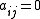 if and only if
if and only if 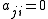
-
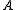 can be written as
can be written as 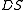 , where
, where 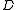 is a diagonal matrixDiagonal matrixIn linear algebra, a diagonal matrix is a matrix in which the entries outside the main diagonal are all zero. The diagonal entries themselves may or may not be zero...
is a diagonal matrixDiagonal matrixIn linear algebra, a diagonal matrix is a matrix in which the entries outside the main diagonal are all zero. The diagonal entries themselves may or may not be zero...
, and is a symmetric matrix.
is a symmetric matrix.
The third condition is not independent but is really a consequence of the first and fourth conditions.
We can always choose a D with positive diagonal entries. In that case,
if
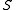 in the above decomposition is positive definite
in the above decomposition is positive definitePositive-definite matrix
In linear algebra, a positive-definite matrix is a matrix that in many ways is analogous to a positive real number. The notion is closely related to a positive-definite symmetric bilinear form ....
, then
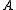 is said to be a Cartan matrix.
is said to be a Cartan matrix.The Cartan matrix of a simple Lie algebra
Lie algebra
In mathematics, a Lie algebra is an algebraic structure whose main use is in studying geometric objects such as Lie groups and differentiable manifolds. Lie algebras were introduced to study the concept of infinitesimal transformations. The term "Lie algebra" was introduced by Hermann Weyl in the...
is the matrix whose elements are the scalar products
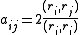
(sometimes called the Cartan integers) where
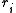 are the simple roots
are the simple rootsRoot system
In mathematics, a root system is a configuration of vectors in a Euclidean space satisfying certain geometrical properties. The concept is fundamental in the theory of Lie groups and Lie algebras...
of the algebra. The entries are integral from one of the properties of root
Root system
In mathematics, a root system is a configuration of vectors in a Euclidean space satisfying certain geometrical properties. The concept is fundamental in the theory of Lie groups and Lie algebras...
s. The first condition follows from the definition, the second from the fact that for
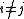 ,
,  is a root which is a linear combination
is a root which is a linear combinationLinear combination
In mathematics, a linear combination is an expression constructed from a set of terms by multiplying each term by a constant and adding the results...
of the simple root
Simple root
in mathematics the term simple root can refer to one of two unrelated notions:*A simple root of a polynomial is a root of multiplicity one*A simple root in a root system is a member of a subset determined by a choice of positive roots...
s ri and rj with a positive coefficient for rj and so, the coefficient for ri has to be nonnegative. The third is true because orthogonality is a symmetric relation. And lastly, let
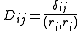 and
and 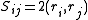 . Because the simple roots span a Euclidean space
. Because the simple roots span a Euclidean spaceEuclidean space
In mathematics, Euclidean space is the Euclidean plane and three-dimensional space of Euclidean geometry, as well as the generalizations of these notions to higher dimensions...
, S is positive definite.
Conversely, given a generalized Cartan matrix, one can recover its corresponding Lie algebra. (See Kac-Moody algebra for more details).
Classification
A matrix A is decomposable if there exists a nonempty proper subset
matrix A is decomposable if there exists a nonempty proper subset 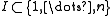 such that
such that 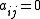 whenever
whenever 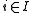 and
and 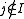 . A is indecomposable if it is not decomposable.
. A is indecomposable if it is not decomposable.Let A be an indecomposable generalized Cartan matrix. We say that A is of finite type if all of its principal minors are positive, that A is of affine type if its proper principal minors are positive and A has determinant
Determinant
In linear algebra, the determinant is a value associated with a square matrix. It can be computed from the entries of the matrix by a specific arithmetic expression, while other ways to determine its value exist as well...
0, and that A is of indefinite type otherwise.
Finite type indecomposable matrices classify the finite dimensional simple Lie algebras (of types
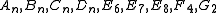 ), while affine type indecomposable matrices classify the affine Lie algebra
), while affine type indecomposable matrices classify the affine Lie algebraAffine Lie algebra
In mathematics, an affine Lie algebra is an infinite-dimensional Lie algebra that is constructed in a canonical fashion out of a finite-dimensional simple Lie algebra. It is a Kac–Moody algebra for which the generalized Cartan matrix is positive semi-definite and has corank 1...
s (say over some algebraically closed field of characteristic 0).
Determinants of the Cartan matrices of the simple Lie algebras
The determinants of the Cartan matrices of the simple Lie algebras given in the following table.  |
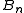 , , 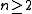 |
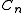 , , 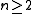 |
 , , 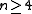 |
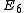 |
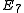 |
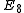 |
 |
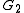 |
| |
|
|
|
|
|
|
|
|
Representations of finite-dimensional algebras
In modular representation theoryModular representation theory
Modular representation theory is a branch of mathematics, and that part of representation theory that studies linear representations of finite group G over a field K of positive characteristic...
, and more generally in the theory of representations of finite-dimensional associative algebra
Associative algebra
In mathematics, an associative algebra A is an associative ring that has a compatible structure of a vector space over a certain field K or, more generally, of a module over a commutative ring R...
s A that are not semisimple
Semisimple algebra
In ring theory, a semisimple algebra is an associative algebra which has trivial Jacobson radical...
, a Cartan matrix is defined by considering a (finite) set of principal indecomposable module
Principal indecomposable module
In mathematics, especially in the area of abstract algebra known as module theory, a principal indecomposable module has many important relations to the study of a ring's modules, especially its simple modules, projective modules, and indecomposable modules....
s and writing composition series
Composition series
In abstract algebra, a composition series provides a way to break up an algebraic structure, such as a group or a module, into simple pieces. The need for considering composition series in the context of modules arises from the fact that many naturally occurring modules are not semisimple, hence...
for them in terms of irreducible modules, yielding a matrix of integers counting the number of occurrences of an irreducible module.
Cartan matrices in M-theory
In M-theoryM-theory
In theoretical physics, M-theory is an extension of string theory in which 11 dimensions are identified. Because the dimensionality exceeds that of superstring theories in 10 dimensions, proponents believe that the 11-dimensional theory unites all five string theories...
, one may consider a geometry with two-cycles
Cycle (mathematics)
In mathematics, and in particular in group theory, a cycle is a permutation of the elements of some set X which maps the elements of some subset S to each other in a cyclic fashion, while fixing all other elements...
which intersects with each other at a finite number of points, at the limit where the area of the two-cycles go to zero. At this limit, there appears a local symmetry group. The matrix of intersection number
Intersection number
In mathematics, and especially in algebraic geometry, the intersection number generalizes the intuitive notion of counting the number of times two curves intersect to higher dimensions, multiple curves, and accounting properly for tangency...
s of a basis of the two-cycles is conjectured to be the Cartan matrix of the Lie algebra
Lie algebra
In mathematics, a Lie algebra is an algebraic structure whose main use is in studying geometric objects such as Lie groups and differentiable manifolds. Lie algebras were introduced to study the concept of infinitesimal transformations. The term "Lie algebra" was introduced by Hermann Weyl in the...
of this local symmetry group http://arxiv.org/abs/hep-th/9707123.
This can be explained as follows. In M-theory one has soliton
Soliton
In mathematics and physics, a soliton is a self-reinforcing solitary wave that maintains its shape while it travels at constant speed. Solitons are caused by a cancellation of nonlinear and dispersive effects in the medium...
s which are two-dimensional surfaces called membranes or 2-branes. A 2-brane has a tension and thus tends to shrink, but it may wrap around a two-cycles which prevents it from shrinking to zero.
One may compactify
Compactification (physics)
In physics, compactification means changing a theory with respect to one of its space-time dimensions. Instead of having a theory with this dimension being infinite, one changes the theory so that this dimension has a finite length, and may also be periodic....
one dimension which is shared by all two-cycles and their intersecting points, and then take the limit where this dimension shrinks to zero, thus getting a dimensional reduction
Dimensional reduction
In physics, a theory in D spacetime dimensions can be redefined in a lower number of dimensions d, by taking all the fields to be independent of the location in the extra D − d dimensions....
over this dimension. Then one gets type IIA string theory
String theory
String theory is an active research framework in particle physics that attempts to reconcile quantum mechanics and general relativity. It is a contender for a theory of everything , a manner of describing the known fundamental forces and matter in a mathematically complete system...
as a limit of M-theory, with 2-branes wrapping a two-cycles now described by an open string stretched between D-brane
D-brane
In string theory, D-branes are a class of extended objects upon which open strings can end with Dirichlet boundary conditions, after which they are named. D-branes were discovered by Dai, Leigh and Polchinski, and independently by Hořava in 1989...
s. There is a U(1) local symmetry group for each D-brane, resembling the degree of freedom
Degrees of freedom (physics and chemistry)
A degree of freedom is an independent physical parameter, often called a dimension, in the formal description of the state of a physical system...
of moving it without changing its orientation. The limit where the two-cycles have zero area is the limit where these D-branes are on top of each other, so that one gets an enhanced local symmetry group.
Now, an open string stretched between two D-branes represents a Lie algebra generator, and the commutator
Commutator
In mathematics, the commutator gives an indication of the extent to which a certain binary operation fails to be commutative. There are different definitions used in group theory and ring theory.-Group theory:...
of two such generator is a third one, represented by an open string which one gets by gluing together the edges of two open strings.
The latter relation between different open strings is dependent on the way 2-branes may intersect in the original M-theory, i.e. in the intersection numbers of two-cycles. Thus the Lie algebra depends entirely on these intersection numbers. The precise relation to the Cartan matrix is because the latter describes the commutators of the simple root
Simple root
in mathematics the term simple root can refer to one of two unrelated notions:*A simple root of a polynomial is a root of multiplicity one*A simple root in a root system is a member of a subset determined by a choice of positive roots...
s, which are related to the two-cycles in the basis that is chosen.
Note that generators in the Cartan subalgebra are represented by open strings which are stretched between a D-brane and itself.

