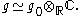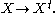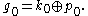Real form (Lie theory)
Encyclopedia
In mathematics
, the notion of a real form relates objects defined over the field of real
and complex
numbers. A real Lie algebra
g0 is called a real form of a complex Lie algebra g if g is the complexification
of g0:
The notion of a real form can also be defined for complex Lie group
s.
Real forms of complex semisimple Lie groups and Lie algebras have been completely classified by Élie Cartan
.
s, the notions of complexification and real form have a natural description in the language of algebraic geometry
.
s, which are obtained from the Dynkin diagram of the complex form by labeling some vertices black (filled), and connecting some other vertices in pairs by arrows, according to certain rules.
It is a basic fact in the structure theory of complex semisimple Lie algebras that every such algebra has two special real forms: one is the compact real form and corresponds to a compact Lie group under the Lie correspondence (its Satake diagram has all vertices blackened), and the other is the split real form and corresponds to a Lie group that is as far as possible from being compact (its Satake diagram has no vertices blackened and no arrows). In the case of the complex special linear group
SL(n,C), the compact real form is the special unitary group
SU(n) and the split real form is the real special linear group SL(n,R). The classification of real forms of semisimple Lie algebras was accomplished by Élie Cartan
in the context of Riemannian symmetric space
s. In general, there may be more than two real forms.
Suppose that g0 is a semisimple Lie algebra over the field of real numbers. By Cartan's criterion, the Killing form is nondegenerate, and can be diagonalized in a suitable basis with the diagonal entries +1 or -1. By Sylvester's law of inertia
, the number of positive entries, or the positive index of intertia, is an invariant of the bilinear form, i.e. it does not depend on the choice of the diagonalizing basis. This is a number between 0 and the dimension of g which is an important invariant of the real Lie algebra, called its index.
g0 = k0 ⊕ p0, the space p0 contains a maximal Abelian subalgebra of g0, i.e. its Cartan subalgebra. Élie Cartan
proved that every complex semisimple Lie algebra g has a split real form, which is unique up to isomorphism. It has maximal index among all real forms.
The split form corresponds to the Satake diagram with no vertices blackened and no arrows.
if the Killing form is negative definite, i.e. the index of g0 is zero. In this case g0 = k0 is a compact Lie algebra
. It is known that under the Lie correspondence, compact Lie algebras correspond to compact Lie groups.
The compact form corresponds to the Satake diagram with all vertices blackened.
Let g0 be a real Lie algebra of matrices over R that is closed under the transpose map,
Then g0 decomposes into the direct sum of its skew-symmetric part
k0 and its symmetric part p0, this is the Cartan decomposition:
The complexification g of g0 decomposes into the direct sum of g0 and ig0. The real vector space of matrices
is a subspace of the complex Lie algebra g that is closed under the commutators and consists of skew-hermitian matrices
. It follows that u0 is a real Lie subalgebra of g, that its Killing form is negative definite (making it a compact Lie algebra), and that the complexification of u0 is g. Therefore, u0 is a compact form of g.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, the notion of a real form relates objects defined over the field of real
Real number
In mathematics, a real number is a value that represents a quantity along a continuum, such as -5 , 4/3 , 8.6 , √2 and π...
and complex
Complex number
A complex number is a number consisting of a real part and an imaginary part. Complex numbers extend the idea of the one-dimensional number line to the two-dimensional complex plane by using the number line for the real part and adding a vertical axis to plot the imaginary part...
numbers. A real Lie algebra
Lie algebra
In mathematics, a Lie algebra is an algebraic structure whose main use is in studying geometric objects such as Lie groups and differentiable manifolds. Lie algebras were introduced to study the concept of infinitesimal transformations. The term "Lie algebra" was introduced by Hermann Weyl in the...
g0 is called a real form of a complex Lie algebra g if g is the complexification
Complexification
In mathematics, the complexification of a real vector space V is a vector space VC over the complex number field obtained by formally extending scalar multiplication to include multiplication by complex numbers. Any basis for V over the real numbers serves as a basis for VC over the complex...
of g0:
The notion of a real form can also be defined for complex Lie group
Lie group
In mathematics, a Lie group is a group which is also a differentiable manifold, with the property that the group operations are compatible with the smooth structure...
s.
Real forms of complex semisimple Lie groups and Lie algebras have been completely classified by Élie Cartan
Élie Cartan
Élie Joseph Cartan was an influential French mathematician, who did fundamental work in the theory of Lie groups and their geometric applications...
.
Real forms for Lie groups and algebraic groups
Using the Lie correspondence between Lie groups and Lie algebras, the notion of a real form can be defined for Lie groups. In the case of linear algebraic groupLinear algebraic group
In mathematics, a linear algebraic group is a subgroup of the group of invertible n×n matrices that is defined by polynomial equations...
s, the notions of complexification and real form have a natural description in the language of algebraic geometry
Algebraic geometry
Algebraic geometry is a branch of mathematics which combines techniques of abstract algebra, especially commutative algebra, with the language and the problems of geometry. It occupies a central place in modern mathematics and has multiple conceptual connections with such diverse fields as complex...
.
Classification
Just as complex semisimple Lie algebras are classified by Dynkin diagrams, the real forms of a semisimple Lie algebra are classified by Satake diagramSatake diagram
In the mathematical study of Lie algebras and Lie groups, a Satake diagram is a generalization of a Dynkin diagram whose configurations classify simple Lie algebras over the field of real numbers...
s, which are obtained from the Dynkin diagram of the complex form by labeling some vertices black (filled), and connecting some other vertices in pairs by arrows, according to certain rules.
It is a basic fact in the structure theory of complex semisimple Lie algebras that every such algebra has two special real forms: one is the compact real form and corresponds to a compact Lie group under the Lie correspondence (its Satake diagram has all vertices blackened), and the other is the split real form and corresponds to a Lie group that is as far as possible from being compact (its Satake diagram has no vertices blackened and no arrows). In the case of the complex special linear group
Special linear group
In mathematics, the special linear group of degree n over a field F is the set of n×n matrices with determinant 1, with the group operations of ordinary matrix multiplication and matrix inversion....
SL(n,C), the compact real form is the special unitary group
Special unitary group
The special unitary group of degree n, denoted SU, is the group of n×n unitary matrices with determinant 1. The group operation is that of matrix multiplication...
SU(n) and the split real form is the real special linear group SL(n,R). The classification of real forms of semisimple Lie algebras was accomplished by Élie Cartan
Élie Cartan
Élie Joseph Cartan was an influential French mathematician, who did fundamental work in the theory of Lie groups and their geometric applications...
in the context of Riemannian symmetric space
Riemannian symmetric space
In differential geometry, representation theory and harmonic analysis, a symmetric space is a smooth manifold whose group of symmetries contains an inversion symmetry about every point. There are two ways to formulate the inversion symmetry, via Riemannian geometry or via Lie theory...
s. In general, there may be more than two real forms.
Suppose that g0 is a semisimple Lie algebra over the field of real numbers. By Cartan's criterion, the Killing form is nondegenerate, and can be diagonalized in a suitable basis with the diagonal entries +1 or -1. By Sylvester's law of inertia
Sylvester's law of inertia
Sylvester's law of inertia is a theorem in matrix algebra about certain properties of the coefficient matrix of a real quadratic form that remain invariant under a change of coordinates...
, the number of positive entries, or the positive index of intertia, is an invariant of the bilinear form, i.e. it does not depend on the choice of the diagonalizing basis. This is a number between 0 and the dimension of g which is an important invariant of the real Lie algebra, called its index.
Split real form
A real form g0 of a complex semisimple Lie algebra g is said to be split, or normal, if in each Cartan decompositionCartan decomposition
The Cartan decomposition is a decomposition of a semisimple Lie group or Lie algebra, which plays an important role in their structure theory and representation theory. It generalizes the polar decomposition of matrices.- Cartan involutions on Lie algebras :...
g0 = k0 ⊕ p0, the space p0 contains a maximal Abelian subalgebra of g0, i.e. its Cartan subalgebra. Élie Cartan
Élie Cartan
Élie Joseph Cartan was an influential French mathematician, who did fundamental work in the theory of Lie groups and their geometric applications...
proved that every complex semisimple Lie algebra g has a split real form, which is unique up to isomorphism. It has maximal index among all real forms.
The split form corresponds to the Satake diagram with no vertices blackened and no arrows.
Compact real form
A real Lie algebra g0 is called compactCompact Lie algebra
In the mathematical field of Lie theory, a Lie algebra is compact if it is the Lie algebra of a compact Lie group. Intrinsically, a compact Lie algebra is a real Lie algebra whose Killing form is negative definite, though this definition does not quite agree with the previous...
if the Killing form is negative definite, i.e. the index of g0 is zero. In this case g0 = k0 is a compact Lie algebra
Compact Lie algebra
In the mathematical field of Lie theory, a Lie algebra is compact if it is the Lie algebra of a compact Lie group. Intrinsically, a compact Lie algebra is a real Lie algebra whose Killing form is negative definite, though this definition does not quite agree with the previous...
. It is known that under the Lie correspondence, compact Lie algebras correspond to compact Lie groups.
The compact form corresponds to the Satake diagram with all vertices blackened.
Construction of the compact real form
In general, the construction of the compact real form uses structure theory of semisimple Lie algebras. For classical Lie algebras there is a more explicit construction.Let g0 be a real Lie algebra of matrices over R that is closed under the transpose map,
Then g0 decomposes into the direct sum of its skew-symmetric part
Skew-symmetric matrix
In mathematics, and in particular linear algebra, a skew-symmetric matrix is a square matrix A whose transpose is also its negative; that is, it satisfies the equation If the entry in the and is aij, i.e...
k0 and its symmetric part p0, this is the Cartan decomposition:
The complexification g of g0 decomposes into the direct sum of g0 and ig0. The real vector space of matrices
is a subspace of the complex Lie algebra g that is closed under the commutators and consists of skew-hermitian matrices
Skew-Hermitian matrix
In linear algebra, a square matrix with complex entries is said to be skew-Hermitian or antihermitian if its conjugate transpose is equal to its negative. That is, the matrix A is skew-Hermitian if it satisfies the relationA^\dagger = -A,\;...
. It follows that u0 is a real Lie subalgebra of g, that its Killing form is negative definite (making it a compact Lie algebra), and that the complexification of u0 is g. Therefore, u0 is a compact form of g.