
Soddy's hexlet
Encyclopedia
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
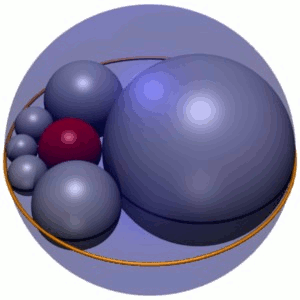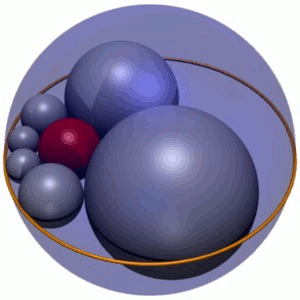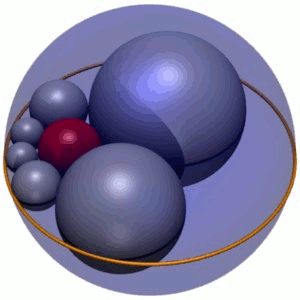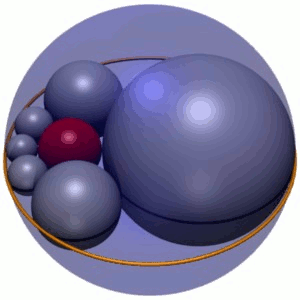
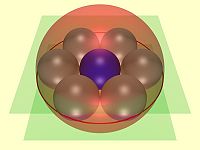
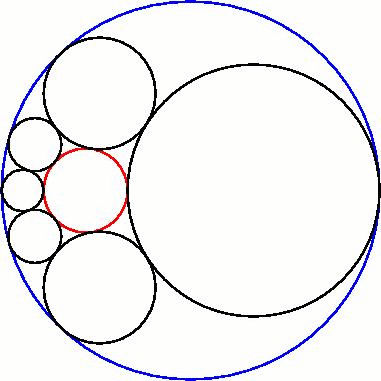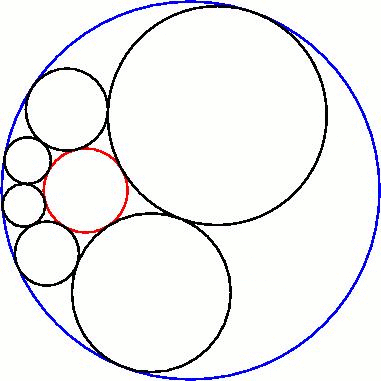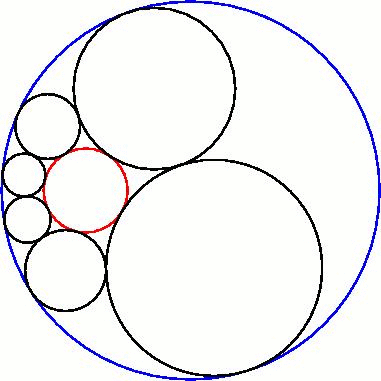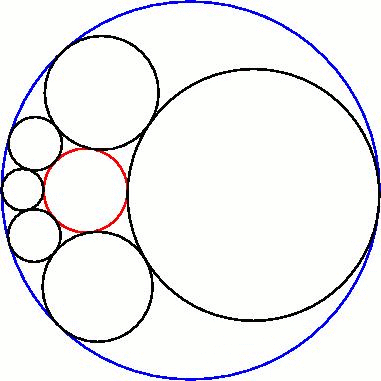
, Soddy's hexlet is a chain of six sphere
Sphere
A sphere is a perfectly round geometrical object in three-dimensional space, such as the shape of a round ball. Like a circle in two dimensions, a perfect sphere is completely symmetrical around its center, with all points on the surface lying the same distance r from the center point...
s (shown in grey in Figure 1), each of which is tangent to both of its neighbors and also to three mutually tangent given spheres. In Figure 1, these three spheres are shown as an outer circumscribing sphere C (blue), and two spheres A and B (green) above and below the plane of their centers. In addition, the hexlet spheres are tangent to a fourth sphere D (red in Figure 1), which is not tangent to the three others.
According to a theorem
Theorem
In mathematics, a theorem is a statement that has been proven on the basis of previously established statements, such as other theorems, and previously accepted statements, such as axioms...
published by Frederick Soddy
Frederick Soddy
Frederick Soddy was an English radiochemist who explained, with Ernest Rutherford, that radioactivity is due to the transmutation of elements, now known to involve nuclear reactions. He also proved the existence of isotopes of certain radioactive elements...
in 1937, it is always possible to find a hexlet for any choice of mutually tangent spheres A, B and C. Indeed, there is an infinite family of hexlets related by rotation and scaling of the hexlet spheres (Figure 1); in this, Soddy's hexlet is the spherical analog of a Steiner chain
Steiner chain
In geometry, a Steiner chain is a set of n circles, all of which are tangent to two given non-intersecting circles , where n is finite and each circle in the chain is tangent to the previous and next circles in the chain...
of six circles. Consistent with Steiner chains, the centers of the hexlet spheres lie in a single plane, on an ellipse. Soddy's hexlet was also discovered independently in Japan, as shown by Sangaku
Sangaku
Sangaku or San Gaku are Japanese geometrical puzzles in Euclidean geometry on wooden tablets which were placed as offerings at Shinto shrines or Buddhist temples during the Edo period by members of all social classes.-History:The Sangaku were painted in color on wooden tablets and hung in the...
tablets from 1822 in the Kanagawa prefecture.
Definition
Soddy's hexlet is a chain of six spheres, labeled S1–S6, each of which is tangent to three given spheres, A, B and C, that are themselves mutually tangent at three distinct points. (For consistency throughout the article, the hexlet spheres will always be depicted in grey, spheres A and B in green, and sphere C in blue.) The hexlet spheres are also tangent to a fourth fixed sphere D (always shown in red) that is not tangent to the three others, A, B and C.Each sphere of Soddy's hexlet is also tangent to its neighbors in the chain; for example, sphere S4 is tangent to S3 and S5. The chain is closed, meaning that every sphere in the chain has two tangent neighbors; in particular, the initial and final spheres, S1 and S6, are tangent to one another.
Annular hexlet
The chain of six spheres can be rotated about the central sphere without affecting their tangencies, showing that there is an infinite family of solutions for this case. As they are rotated, the spheres of the hexlet trace out a torus
Torus
In geometry, a torus is a surface of revolution generated by revolving a circle in three dimensional space about an axis coplanar with the circle...
(a doughnut-shaped surface); in other words, a torus is the envelope
Envelope (mathematics)
In geometry, an envelope of a family of curves in the plane is a curve that is tangent to each member of the family at some point. Classically, a point on the envelope can be thought of as the intersection of two "adjacent" curves, meaning the limit of intersections of nearby curves...
of this family of hexlets.
Solution by inversion
The general problem of finding a hexlet for three given mutually tangent spheres A, B and C can be reduced to the annular case using inversion. This geometrical operation always transforms spheres into spheres or into planes, which may be regarded as spheres of infinite radius. A sphere is transformed into a plane if and only if the sphere passes through the center of inversion. An advantage of inversion is that it preserves tangency; if two spheres are tangent before the transformation, they remain so after. Thus, if the inversion transformation is chosen judiciously, the problem can be reduced to a simpler case, such as the annular Soddy's hexlet. Inversion is reversible; repeating an inversion in the same point returns the transformed objects to their original size and position.Inversion in the point of tangency between spheres A and B transforms them into parallel planes, which may be denoted as a and b. Since sphere C is tangent to both A and B and does not pass through the center of inversion, C is transformed into another sphere c that is tangent to both planes; hence, c is sandwiched between the two planes a and b. This is the annular Soddy's hexlet (Figure 2). Six spheres s1–s6 may be packed around c and likewise sandwiched between the bounding planes a and b. Re-inversion restores the three original spheres, and transforms s1–s6 into a hexlet for the original problem. In general, these hexlet spheres S1–S6 have different radii.
An infinite variety of hexlets may be generated by rotating the six balls s1–s6 in their plane by an arbitrary angle before re-inverting them. The envelope produced by such rotations is the torus
Torus
In geometry, a torus is a surface of revolution generated by revolving a circle in three dimensional space about an axis coplanar with the circle...
that surrounds the sphere c and is sandwiched between the two planes a and b; thus, the torus has an inner radius r and outer radius 3r. After the re-inversion, this torus becomes a Dupin cyclide (Figure 3).

Dupin cyclide
The envelopeEnvelope (mathematics)
In geometry, an envelope of a family of curves in the plane is a curve that is tangent to each member of the family at some point. Classically, a point on the envelope can be thought of as the intersection of two "adjacent" curves, meaning the limit of intersections of nearby curves...
of Soddy's hexlets is a Dupin cyclide, an inversion of the torus
Torus
In geometry, a torus is a surface of revolution generated by revolving a circle in three dimensional space about an axis coplanar with the circle...
. Thus Soddy's construction shows that a cyclide of Dupin is the envelope of a 1-parameter family of spheres in two different ways, and each sphere in either family is tangent to two spheres in same family and three spheres in the other family. This result was probably known to Charles Dupin
Charles Dupin
Pierre Charles François Dupin was a French Catholic mathematician.He studied geometry with Monge at the École Polytechnique and then became a naval engineer. In 1819 he was appointed professor at the Conservatoire des Arts et Métiers; he kept this post until 1854...
, who discovered the cyclides that bear his name in his 1803 dissertation under Gaspard Monge
Gaspard Monge
Gaspard Monge, Comte de Péluse was a French mathematician, revolutionary, and was inventor of descriptive geometry. During the French Revolution, he was involved in the complete reorganization of the educational system, founding the École Polytechnique...
.
Relation to Steiner chains
Steiner chain
In geometry, a Steiner chain is a set of n circles, all of which are tangent to two given non-intersecting circles , where n is finite and each circle in the chain is tangent to the previous and next circles in the chain...
of six circles.
Parabolic and hyperbolic hexlets
It is assumed that spheres A and B are the same size.In any elliptic
Ellipse
In geometry, an ellipse is a plane curve that results from the intersection of a cone by a plane in a way that produces a closed curve. Circles are special cases of ellipses, obtained when the cutting plane is orthogonal to the cone's axis...
hexlet, such as the one shown at the top of the article, there are two tangent planes to the hexlet. In order for an elliptic hexlet to exist, the radius of C must be less than one quarter that of A. If C's radius is one quarter of A's, each sphere will become a plane in the journey. The inverted image shows a normal elliptic hexlet, though, and in the parabolic
Parabola
In mathematics, the parabola is a conic section, the intersection of a right circular conical surface and a plane parallel to a generating straight line of that surface...
hexlet, the point where a sphere turns into a plane is precisely when its inverted image passes through the centre of inversion. In such a hexlet there is only one tangent plane to the hexlet. The line of the centres of a parabolic hexlet is a parabola.
If C is even larger than that, a hyperbolic
Hyperbola
In mathematics a hyperbola is a curve, specifically a smooth curve that lies in a plane, which can be defined either by its geometric properties or by the kinds of equations for which it is the solution set. A hyperbola has two pieces, called connected components or branches, which are mirror...
hexlet is formed, and now there are no tangent planes at all. Label the spheres S1 to S6. S1 thus cannot go very far until it becomes a plane (where its inverted image passes through the centre of inversion) and then reverses its concavity (where its inverted image surrounds the centre of inversion). Now the line of the centres is a hyperbola.
The limiting case is when A, B and C are all the same size. The hexlet now becomes straight. S1 is small as it passes through the hole between A, B and C, and grows till it becomes a plane tangent to them. The centre of inversion is now also with a point of tangency with the image of S6, so it is also a plane tangent to A, B and C. As S1 proceeds, its concavity is reversed and now it surrounds all the other spheres, tangent to A, B, C, S2 and S6. S2 pushes upwards and grows to become a tangent plane and S6 shrinks. S1 then obtains S6's former position as a tangent plane. It then reverses concavity again and passes through the hole again, beginning another round trip. Now the line of centres is a degenerate
Degeneracy (mathematics)
In mathematics, a degenerate case is a limiting case in which a class of object changes its nature so as to belong to another, usually simpler, class....
hyperbola, where it has collapsed into two straight lines.
Sangaku tablets
The Japanese mathematicians analized the packing problems which circles and polygons, balls and polyhedrons contact and often found the theorems independently before the West mathematicians discovery. The SangakuSangaku
Sangaku or San Gaku are Japanese geometrical puzzles in Euclidean geometry on wooden tablets which were placed as offerings at Shinto shrines or Buddhist temples during the Edo period by members of all social classes.-History:The Sangaku were painted in color on wooden tablets and hung in the...
about hexlet was made by Irisawa Shintarô Hiroatsu in the family of Uchida Itsumi and dedicated to Samukawa Shrine
Samukawa Shrine
is a Shinto shrine in the town of Samukawa in Kanagawa Prefecture, Japan. The shrine is located on the bank of the Sagami River, about eight kilometers from the river’s mouth.-History:...
on May, 1822. The original sangaku has been lost and recorded in the Uchida's book of Kokinsankagami on 1832. The replica of the sangaku was made from the record and dedicated to Hôtoku museum in Samukawa Shrine on August, 2009.
The sangaku by Irisawa consists of 3 problems and the third problem relates to Soddy's hexlet: "the diameter of the outer circumscribing sphere is 30 sun (unit)
Cun
Cun may refer to:* Cun , a traditional Chinese unit of measure* Villages of China * Cún, a village in HungaryCUN may refer to:* Cancún International Airport, IATA code: CUN...
. The diameter of the nucleus balls are 10 sun and 6 sun each other. The diameter of one ball of the chain balls is 5 sun. Then I asked it the diameters of the remaining balls. The answer is 15 sun, 10 sun, 3.75 sun, 2.5 sun and 2+8/11 sun."
By his answer, the method to calculate the diameters of the balls is written down and can consider it the following formulas to be given in the modern scale. If the ratio of the diameter of the outside ball to the nucleus balls are a1, a2, and if the ratio of the diameter to the chain balls are c1, ..., c6. I want to represent c2, ..., c6 by a1, a2, c1. If

then,
 .
.Then c1 + c4 = c2 + c5 = c3 + c6. If r1, ..., r6 are the diameters of six balls, then we get the formula:
-
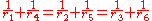 .
.
External links
- Japanese Temple Geometry - The animation 0 of SANGAKU PROBLEM 0 shows the case which the radiuses of spheres A and B are equal each other and the centers of spheres A, B and C are on the line. The animation 1 shows the case which the radiuses of spheres A and B are equal each other and the centers of spheres A, B and C are not on the line. The animation 2 shows the case which the radiuses of spheres A and B are not equal each other. The animation 3 shows the case which the centers of spheres A, B and C are on the line and the radiuses of spheres A and B are variable.
- Replica of Sangaku at Hôtoku museum in Samukawa Shrine - The third problem relates to Soddy's hexlet.

