
Entanglement witness
Encyclopedia
In quantum information theory, an entanglement witness is an object of geometric nature which distinguishes an entangled state
from separable ones.
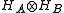 . A mixed state
. A mixed state
ρ is then a trace-class positive operator on the state space which has trace 1. We can view the family of states as a subset of the real Banach space
generated by the Hermitian trace-class operators, with the trace norm. A mixed state ρ is separable if it can be approximated, in the trace norm, by states of the form
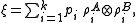
where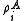 's and
's and  's are pure states on the subsystems A and B respectively. So the family of separable states is the closed convex hull
's are pure states on the subsystems A and B respectively. So the family of separable states is the closed convex hull
of pure product states. We will make use of the following variant of Hahn–Banach theorem
:
Theorem Let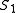 and
and  be convex closed sets in a real Banach space and one of them is compact
be convex closed sets in a real Banach space and one of them is compact
, then there exists a bounded functional
f separating the two sets.
This is a generalization of the fact that, in real Euclidean space, given a convex set and a point outside, there always exists an affine subspace separating the two. The affine subspace manifests itself as the functional f. In the present context, the family of separable states is a convex set in the space of trace class operators. If ρ is an entangled state (thus lying outside the convex set), then by theorem above, there is a functional f separating ρ from the separable states. It is this functional f, or its identification as an operator, that we call an entanglement witness. There are more than one hyperplane separating a closed convex set and a point lying outside of it. So for an entangled state there are more than one entanglement witnesses. Recall the fact that the dual space of the Banach space of trace-class operators is isomorphic to the set of bounded operator
s. Therefore we can identify f with a Hermitian operator A. Therefore, modulo a few details, we have shown the existence of an entanglement witness given an entangled state:
Theorem For every entangled state ρ, there exists a Hermitian operator A such that
 , and
, and  for all separable states σ.
for all separable states σ.
When both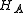 and
and 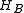 have finite dimension, there is no difference between trace-class and Hilbert–Schmidt operators. So in that case A can be given by Riesz representation theorem
have finite dimension, there is no difference between trace-class and Hilbert–Schmidt operators. So in that case A can be given by Riesz representation theorem
. As an immediate corollary, we have:
Theorem A mixed state σ is separable if and only if
for any bounded operator A satisfying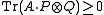 , for all product pure state
, for all product pure state 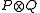 .
.
If a state is separable, clearly the desired implication from the theorem must hold. On the other hand, given an entangled state, one of its entanglement witnesses will violate the given condition.
Thus if a bounded functional f of the trace-class Banach space and f is positive on the product pure states, then f, or its identification as a Hermitian operator, is an entanglement witness. Such a f indicates the entanglement of some state.
Using the isomorphism between entanglement witnesses and non-completely positive maps, it was shown (by the Horodecki's) that
Theorem A mixed state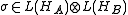 is separable if for every positive map Λ from bounded operators on
is separable if for every positive map Λ from bounded operators on  to bounded operators on
to bounded operators on 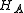 , the operator
, the operator 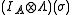 is positive, where
is positive, where 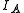 is the identity map on
is the identity map on  , the bounded operators on
, the bounded operators on  .
.
Quantum entanglement
Quantum entanglement occurs when electrons, molecules even as large as "buckyballs", photons, etc., interact physically and then become separated; the type of interaction is such that each resulting member of a pair is properly described by the same quantum mechanical description , which is...
from separable ones.
Details
Let a composite quantum system have state space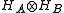 . A mixed state
. A mixed stateDensity matrix
In quantum mechanics, a density matrix is a self-adjoint positive-semidefinite matrix of trace one, that describes the statistical state of a quantum system...
ρ is then a trace-class positive operator on the state space which has trace 1. We can view the family of states as a subset of the real Banach space
Banach space
In mathematics, Banach spaces is the name for complete normed vector spaces, one of the central objects of study in functional analysis. A complete normed vector space is a vector space V with a norm ||·|| such that every Cauchy sequence in V has a limit in V In mathematics, Banach spaces is the...
generated by the Hermitian trace-class operators, with the trace norm. A mixed state ρ is separable if it can be approximated, in the trace norm, by states of the form
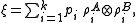
where
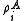 's and
's and  's are pure states on the subsystems A and B respectively. So the family of separable states is the closed convex hull
's are pure states on the subsystems A and B respectively. So the family of separable states is the closed convex hullConvex hull
In mathematics, the convex hull or convex envelope for a set of points X in a real vector space V is the minimal convex set containing X....
of pure product states. We will make use of the following variant of Hahn–Banach theorem
Hahn–Banach theorem
In mathematics, the Hahn–Banach theorem is a central tool in functional analysis. It allows the extension of bounded linear functionals defined on a subspace of some vector space to the whole space, and it also shows that there are "enough" continuous linear functionals defined on every normed...
:
Theorem Let
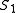 and
and  be convex closed sets in a real Banach space and one of them is compact
be convex closed sets in a real Banach space and one of them is compactCompact space
In mathematics, specifically general topology and metric topology, a compact space is an abstract mathematical space whose topology has the compactness property, which has many important implications not valid in general spaces...
, then there exists a bounded functional
Linear functional
In linear algebra, a linear functional or linear form is a linear map from a vector space to its field of scalars. In Rn, if vectors are represented as column vectors, then linear functionals are represented as row vectors, and their action on vectors is given by the dot product, or the...
f separating the two sets.
This is a generalization of the fact that, in real Euclidean space, given a convex set and a point outside, there always exists an affine subspace separating the two. The affine subspace manifests itself as the functional f. In the present context, the family of separable states is a convex set in the space of trace class operators. If ρ is an entangled state (thus lying outside the convex set), then by theorem above, there is a functional f separating ρ from the separable states. It is this functional f, or its identification as an operator, that we call an entanglement witness. There are more than one hyperplane separating a closed convex set and a point lying outside of it. So for an entangled state there are more than one entanglement witnesses. Recall the fact that the dual space of the Banach space of trace-class operators is isomorphic to the set of bounded operator
Bounded operator
In functional analysis, a branch of mathematics, a bounded linear operator is a linear transformation L between normed vector spaces X and Y for which the ratio of the norm of L to that of v is bounded by the same number, over all non-zero vectors v in X...
s. Therefore we can identify f with a Hermitian operator A. Therefore, modulo a few details, we have shown the existence of an entanglement witness given an entangled state:
Theorem For every entangled state ρ, there exists a Hermitian operator A such that
 , and
, and  for all separable states σ.
for all separable states σ.When both
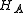 and
and 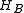 have finite dimension, there is no difference between trace-class and Hilbert–Schmidt operators. So in that case A can be given by Riesz representation theorem
have finite dimension, there is no difference between trace-class and Hilbert–Schmidt operators. So in that case A can be given by Riesz representation theoremRiesz representation theorem
There are several well-known theorems in functional analysis known as the Riesz representation theorem. They are named in honour of Frigyes Riesz.- The Hilbert space representation theorem :...
. As an immediate corollary, we have:
Theorem A mixed state σ is separable if and only if

for any bounded operator A satisfying
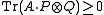 , for all product pure state
, for all product pure state 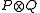 .
.If a state is separable, clearly the desired implication from the theorem must hold. On the other hand, given an entangled state, one of its entanglement witnesses will violate the given condition.
Thus if a bounded functional f of the trace-class Banach space and f is positive on the product pure states, then f, or its identification as a Hermitian operator, is an entanglement witness. Such a f indicates the entanglement of some state.
Using the isomorphism between entanglement witnesses and non-completely positive maps, it was shown (by the Horodecki's) that
Theorem A mixed state
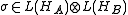 is separable if for every positive map Λ from bounded operators on
is separable if for every positive map Λ from bounded operators on  to bounded operators on
to bounded operators on 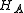 , the operator
, the operator 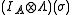 is positive, where
is positive, where 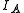 is the identity map on
is the identity map on  , the bounded operators on
, the bounded operators on  .
.

