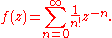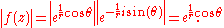Weierstrass–Casorati theorem
Encyclopedia
In complex analysis
, a branch of mathematics, the Casorati–Weierstrass theorem describes the behaviour of meromorphic function
s near essential singularities
. It is named for Karl Theodor Wilhelm Weierstrass and Felice Casorati
.
U in the complex plane
containing the number z0, and a function f that is holomorphic
on U \ {z0}, but has an essential singularity
at z0 . The Casorati–Weierstrass theorem then states that
This can also be stated as follows:
Or in still more descriptive terms:
This form of the theorem also applies if f is only meromorphic.
The theorem is considerably strengthened by Picard's great theorem, which states, in the notation above, that f assumes every complex value, with one possible exception, infinitely often on V.
(1/z) has an essential singularity at z0 = 0, but the function g(z) = 1/z3 does not (it has a pole at 0).
Consider the function
This function has the following Laurent series
about the essential singular point
at z0:
Because
exists for all points z ≠ 0 we know that ƒ(z) is analytic in the punctured neighborhood of z = 0. Hence it is an isolated singularity, as well as being an essential singularity
.
Using a change of variable to polar coordinates
our function, ƒ(z) = e1/z becomes:
Taking the absolute value
of both sides:
Thus, for values of θ such that cos θ > 0, we have
as 
, and for 
, 
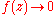as 
.
Consider what happens, for example when z takes values on a circle of diameter 1/R tangent to the imaginary axis. This circle is given by r = (1/R) cos θ. Then,
and
Thus,
may take any positive value other than zero by the appropriate choice of R. As 
on the circle, 
with R fixed. So this part of the equation:
takes on all values on the unit circle
infinitely often. Hence f(z) takes on the value of every number in the complex plane
except for zero infinitely often.
Take as given that function f is meromorphic
on some punctured neighborhood V \ {z0}, and that z0 is an essential singularity. Assume by way of contradiction that some value b exists that the function can never get close to; that is: assume that there is some complex value b and some ε > 0 such that |f(z) − b| ≥ ε for all z in V at which f is defined.
Then the new function:
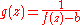
must be holomorphic on V \ {z0}, with zeroes
at the poles of f, and bounded by 1/ε. It can therefore be analytically continued (or continuously extended, or holomorphically extended) to all of V by Riemann's analytic continuation theorem. So the original function can be expressed in terms of g:
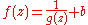
for all arguments z in V \ {z0}. Consider the two possible cases for
If the limit is 0, then f has a pole at z0 . If the limit is not 0, then z0 is a removable singularity of f . Both possibilities contradict the assumption that the point z0 is an essential singularity
of the function f . Hence the assumption is false and the theorem holds.
Complex analysis
Complex analysis, traditionally known as the theory of functions of a complex variable, is the branch of mathematical analysis that investigates functions of complex numbers. It is useful in many branches of mathematics, including number theory and applied mathematics; as well as in physics,...
, a branch of mathematics, the Casorati–Weierstrass theorem describes the behaviour of meromorphic function
Meromorphic function
In complex analysis, a meromorphic function on an open subset D of the complex plane is a function that is holomorphic on all D except a set of isolated points, which are poles for the function...
s near essential singularities
Essential singularity
In complex analysis, an essential singularity of a function is a "severe" singularity near which the function exhibits extreme behavior.The category essential singularity is a "left-over" or default group of singularities that are especially unmanageable: by definition they fit into neither of the...
. It is named for Karl Theodor Wilhelm Weierstrass and Felice Casorati
Felice Casorati (mathematician)
Felice Casorati was an Italian mathematician best known for the Casorati-Weierstrass theorem in complex analysis...
.
Formal statement of the theorem
Start with some open subsetOpen set
The concept of an open set is fundamental to many areas of mathematics, especially point-set topology and metric topology. Intuitively speaking, a set U is open if any point x in U can be "moved" a small amount in any direction and still be in the set U...
U in the complex plane
Complex number
A complex number is a number consisting of a real part and an imaginary part. Complex numbers extend the idea of the one-dimensional number line to the two-dimensional complex plane by using the number line for the real part and adding a vertical axis to plot the imaginary part...
containing the number z0, and a function f that is holomorphic
Holomorphic function
In mathematics, holomorphic functions are the central objects of study in complex analysis. A holomorphic function is a complex-valued function of one or more complex variables that is complex differentiable in a neighborhood of every point in its domain...
on U \ {z0}, but has an essential singularity
Essential singularity
In complex analysis, an essential singularity of a function is a "severe" singularity near which the function exhibits extreme behavior.The category essential singularity is a "left-over" or default group of singularities that are especially unmanageable: by definition they fit into neither of the...
at z0 . The Casorati–Weierstrass theorem then states that
- if V is any neighbourhoodNeighbourhood (mathematics)In topology and related areas of mathematics, a neighbourhood is one of the basic concepts in a topological space. Intuitively speaking, a neighbourhood of a point is a set containing the point where you can move that point some amount without leaving the set.This concept is closely related to the...
of z0 contained in U, then f(V \ {z0}) is denseDense setIn topology and related areas of mathematics, a subset A of a topological space X is called dense if any point x in X belongs to A or is a limit point of A...
in C.
This can also be stated as follows:
- for any ε > 0, δ >0, and complex number w, there exists a complex number z in U with |z − z0| < δ and |f(z) − w| < ε .
Or in still more descriptive terms:
- f comes arbitrarily close to any complex value in every neighbourhood of z0.
This form of the theorem also applies if f is only meromorphic.
The theorem is considerably strengthened by Picard's great theorem, which states, in the notation above, that f assumes every complex value, with one possible exception, infinitely often on V.
Examples
The function f(z) = expExponential function
In mathematics, the exponential function is the function ex, where e is the number such that the function ex is its own derivative. The exponential function is used to model a relationship in which a constant change in the independent variable gives the same proportional change In mathematics,...
(1/z) has an essential singularity at z0 = 0, but the function g(z) = 1/z3 does not (it has a pole at 0).
Consider the function
This function has the following Laurent series
Laurent series
In mathematics, the Laurent series of a complex function f is a representation of that function as a power series which includes terms of negative degree. It may be used to express complex functions in cases where...
about the essential singular point
Essential singularity
In complex analysis, an essential singularity of a function is a "severe" singularity near which the function exhibits extreme behavior.The category essential singularity is a "left-over" or default group of singularities that are especially unmanageable: by definition they fit into neither of the...
at z0:
Because
exists for all points z ≠ 0 we know that ƒ(z) is analytic in the punctured neighborhood of z = 0. Hence it is an isolated singularity, as well as being an essential singularityEssential singularity
In complex analysis, an essential singularity of a function is a "severe" singularity near which the function exhibits extreme behavior.The category essential singularity is a "left-over" or default group of singularities that are especially unmanageable: by definition they fit into neither of the...
.
Using a change of variable to polar coordinates
our function, ƒ(z) = e1/z becomes:
Taking the absolute value
Absolute value
In mathematics, the absolute value |a| of a real number a is the numerical value of a without regard to its sign. So, for example, the absolute value of 3 is 3, and the absolute value of -3 is also 3...
of both sides:
Thus, for values of θ such that cos θ > 0, we have
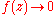as , and for , as .Consider what happens, for example when z takes values on a circle of diameter 1/R tangent to the imaginary axis. This circle is given by r = (1/R) cos θ. Then,
and
Thus,
may take any positive value other than zero by the appropriate choice of R. As on the circle, with R fixed. So this part of the equation:
takes on all values on the unit circle
Unit circle
In mathematics, a unit circle is a circle with a radius of one. Frequently, especially in trigonometry, "the" unit circle is the circle of radius one centered at the origin in the Cartesian coordinate system in the Euclidean plane...
infinitely often. Hence f(z) takes on the value of every number in the complex plane
Complex plane
In mathematics, the complex plane or z-plane is a geometric representation of the complex numbers established by the real axis and the orthogonal imaginary axis...
except for zero infinitely often.
Proof of the theorem
A short proof of the theorem is as follows:Take as given that function f is meromorphic
Meromorphic function
In complex analysis, a meromorphic function on an open subset D of the complex plane is a function that is holomorphic on all D except a set of isolated points, which are poles for the function...
on some punctured neighborhood V \ {z0}, and that z0 is an essential singularity. Assume by way of contradiction that some value b exists that the function can never get close to; that is: assume that there is some complex value b and some ε > 0 such that |f(z) − b| ≥ ε for all z in V at which f is defined.
Then the new function:
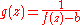
must be holomorphic on V \ {z0}, with zeroes
Zero (complex analysis)
In complex analysis, a zero of a holomorphic function f is a complex number a such that f = 0.-Multiplicity of a zero:A complex number a is a simple zero of f, or a zero of multiplicity 1 of f, if f can be written asf=g\,where g is a holomorphic function g such that g is not zero.Generally, the...
at the poles of f, and bounded by 1/ε. It can therefore be analytically continued (or continuously extended, or holomorphically extended) to all of V by Riemann's analytic continuation theorem. So the original function can be expressed in terms of g:
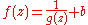
for all arguments z in V \ {z0}. Consider the two possible cases for
If the limit is 0, then f has a pole at z0 . If the limit is not 0, then z0 is a removable singularity of f . Both possibilities contradict the assumption that the point z0 is an essential singularity
Essential singularity
In complex analysis, an essential singularity of a function is a "severe" singularity near which the function exhibits extreme behavior.The category essential singularity is a "left-over" or default group of singularities that are especially unmanageable: by definition they fit into neither of the...
of the function f . Hence the assumption is false and the theorem holds.