Burgers vector
Encyclopedia
The Burgers vector, named after Dutch physicist Jan Burgers, is a vector, often denoted b, that represents the magnitude and direction of the lattice distortion of dislocation
in a crystal lattice
.
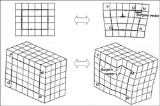
The vector's magnitude and direction is best understood when the dislocation-bearing crystal structure is first visualized without the dislocation, that is, the perfect crystal structure. In this perfect crystal
structure, a rectangle whose lengths and widths are integer multiples of "a" (the unit cell length) is drawn encompassing the site of the original dislocation's origin. Once this encompassing rectangle is drawn, the dislocation can be introduced. This dislocation will have the effect of deforming, not only the perfect crystal structure, but the rectangle as well. Said rectangle could have one of its sides disjoined from the perpendicular side, severing the connection of the length and width line segments of the rectangle at one of the rectangle's corners, and displacing each line segment
from each other. What was once a rectangle before the dislocation was introduced is now an open geometric figure, whose opening defines the direction and magnitude of the Burgers vector. Specifically, the breadth of the opening defines the magnitude of the Burgers vector, and, when a set of fixed coordinates is introduced, an angle between the termini of the dislocated rectangle's length line segment and width line segment may be specified.
The direction of the vector depends on the plane of dislocation, which is usually on the closest-packed plane of unit cell.
The magnitude is usually represented by equation:
Dislocation
In materials science, a dislocation is a crystallographic defect, or irregularity, within a crystal structure. The presence of dislocations strongly influences many of the properties of materials...
in a crystal lattice
Crystal structure
In mineralogy and crystallography, crystal structure is a unique arrangement of atoms or molecules in a crystalline liquid or solid. A crystal structure is composed of a pattern, a set of atoms arranged in a particular way, and a lattice exhibiting long-range order and symmetry...
.
Perfect crystal
Crystalline materials are made up of solid regions of ordered matter . These regions are known as crystals. A perfect crystal is one that contains no point, linear, or planar imperfections...
structure, a rectangle whose lengths and widths are integer multiples of "a" (the unit cell length) is drawn encompassing the site of the original dislocation's origin. Once this encompassing rectangle is drawn, the dislocation can be introduced. This dislocation will have the effect of deforming, not only the perfect crystal structure, but the rectangle as well. Said rectangle could have one of its sides disjoined from the perpendicular side, severing the connection of the length and width line segments of the rectangle at one of the rectangle's corners, and displacing each line segment
Line segment
In geometry, a line segment is a part of a line that is bounded by two end points, and contains every point on the line between its end points. Examples of line segments include the sides of a triangle or square. More generally, when the end points are both vertices of a polygon, the line segment...
from each other. What was once a rectangle before the dislocation was introduced is now an open geometric figure, whose opening defines the direction and magnitude of the Burgers vector. Specifically, the breadth of the opening defines the magnitude of the Burgers vector, and, when a set of fixed coordinates is introduced, an angle between the termini of the dislocated rectangle's length line segment and width line segment may be specified.
The direction of the vector depends on the plane of dislocation, which is usually on the closest-packed plane of unit cell.
The magnitude is usually represented by equation:
-
where a is the unit cell length of the crystal, ||b|| is the magnitude of Burgers vector and h, k, and l are the components of Burgers vector, b =. In most metallic materials, the magnitude of the Burgers vector for a dislocation is of a magnitude equal to the interatomic spacing of the material, since a single dislocation will offset the crystal lattice by one close-packed crystallographic spacing unit.
In edge dislocations, the Burgers vector and dislocation line are at right angles to one another. In screw dislocations, they are parallel.
The Burgers vector is significant in determining the yield strengthYield (engineering)The yield strength or yield point of a material is defined in engineering and materials science as the stress at which a material begins to deform plastically. Prior to the yield point the material will deform elastically and will return to its original shape when the applied stress is removed...
of a material by affecting solute hardeningSolid solution strengtheningSolid solution strengthening is a type of alloying that can be used to improve the strength of a pure metal. The technique works by adding atoms of one element to the crystalline lattice of another element . The alloying element diffuses into the matrix, forming a solid solution...
, precipitation hardening and work hardeningWork hardeningWork hardening, also known as strain hardening or cold working, is the strengthening of a metal by plastic deformation. This strengthening occurs because of dislocation movements within the crystal structure of the material. Any material with a reasonably high melting point such as metals and...
.
In materials science/engineering it is often useful to know the magnitude of the Burger’s vector in metres. This is easily done for BCC and FCC lattice materials using the previously mentioned equation as only the slip system and unit cell length 'a' need to be known. So for a FCC lattice where a = 2 R (2)^1/2 and the slip system is <110> the length of the Burger’s vector is simply b = 2 R, where R is the atomic radius. The HCP system is even simpler with the Burger’s vector simply equal to the unit cell length a, I.E. b =a, where a = 2 R for the HCP system. Hence the Burger’s vector of titanium would be 0.29 nm long.